Digital seismic recordings in this frequency band always contain noise, which essentially can be attributed to ambient Earth noise and instrumental noise, or self-noise, of the recording system.
Instrumental noise of today's seismic sensors and high resolution digitizers is usually not considered during the interpretation of seismic data. However, this type of noise may dominate at lower frequencies (e.g. below 0.02 Hz) or at higher frequencies at sites with very low ambient Earth noise levels. In cooperation with Utrecht University (Faculty of Geosciences) KNMI developed a new method to measure instrumental noise in seismic recording systems using ambient Earth noise recordings1). This new method extracts self-noise and relative transfer functions of the recording systems from the measurements only, and does not require a priori information about the transfer function of each channel. As a consequence the method reveals under which conditions the interpretation of data may be biased by the recording system.
Ambient Earth noise, or background noise, is defined as seismic signals in the absence of transient signals from earthquakes. The U.S. Geological Survey New Low Noise Model (NLNM)2) is often used as reference model for background noise. This model was constructed from a large number of vertical seismograms from many globally distributed seismic stations, and represents the lowermost envelope of a large set of power spectral densities of vertical true ground acceleration over the entire seismic frequency band. Essentially, this envelope reflects the minimum seismic noise level which is always expected in seismic recordings. The NLNM model is often used for the purpose of site selection or to compare the quality of seismic sites in terms of noise. Figure 1 displays the probability density functions of the background noise3) in The Netherlands, recorded at three seismic stations.
Many of the large features of the NLNM are well understood, and the ambient Earth noise sources may roughly be classified in the frequency domain as follows:
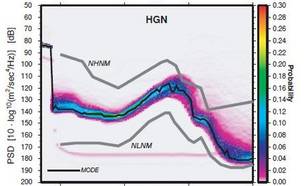
Not only for site selection purposes the NLNM model is important, but it also has been proven to be useful in seismic network monitoring purposes, e.g. for the Virtual European Broadband Seismograph Network (VEBSN)8), and in monitoring volcanic activity9). Such seismic networks continuously monitor the power spectral density of the background noise and compare these at specific frequencies with the NLNM to detect sudden changes or anomalies. These may, for example, indicate an increase of seismic activity, changes in site conditions or instrumental problems. KNMI seismic stations HGN, WIT and WTSB in The Netherlands, as well as the KNMI seismic stations on the Netherlands Antilles (Saba, St. Eustatius and St. Maarten) are continuously monitored in this way. Figure 3 is an example showing the ambient noise variation in time.
Below a few mHz the seismic noise correlates with local barometric pressure variations, mainly due to gravitational attraction by atmospheric masses above the seismic station. Correcting the vertical seismic recordings below a few mHz for atmospheric pressure changes permits the achievement of noise levels even below the NLNM4,10), which means that the NLNM may need some minor revision. Also a new analysis technique11) applied to recordings from the Global Seismographic Network (GSN) shows noise levels below the NLNM. The interpretation of such low noise data would only makes sense if the noise level of the data is above the noise levels of sensor and digitizer at these low frequencies. It is for these reasons that accurate knowledge or self-noise of the seismic equipment is crucial, and a new technique to estimate instrumental self-noise was developed.
Broadband and very broadband seismic sensors are used in global, regional and even local seismological studies, because of their wide frequency bandwidth, large dynamic range and low self-noise level. The bandwidth of the sensor specifies the frequency range in which the instrument has a more or less flat response to ground velocity, and may cover more than 4 logarithmic frequency decades. Today's broadband seismometers are of a force balance feedback system to provide a dynamic range up to about 160 dB, to capture signals from ambient Earth noise to earthquakes of magnitude 9.5 at 90 degrees epicentral distance. At low frequencies instrumental noise of today’s seismic sensors and dataloggers is of inherent 1/f type of noise, noise whose power spectral density is inversely proportional to frequency. The self-noise for present seismic systems is typically close to the NLNM over an extended bandwidth, from a few hundred seconds to a few Hz, and probably defines the NLNM at low frequencies.
In the conventional approach to estimate the self-noise of linear systems, two systems are used and fed by a common, coherent input signal. This technique has been used in many studies12), also to calibrate seismometers13), in which two seismometers are placed close together so that it can be assumed they record the same ground motion. The mathematical solution of such a system is very simple, but the practical application is limited because the method assumes that one of the pair of sensors has an accurately known frequency response. Small errors in the transfer functions (or gains) in the two linear systems will cause relatively large errors in the calculated noise levels14). Our approach1)uses three linear systems which are also fed by a common input signal. The mathematical solution for this system does not need a priori information about the frequency response of each system. The only assumptions are that (1) the self noise between each pair of 2 systems is uncorrelated and (2) the self noise and the input signal are uncorrelated. The mathematical description of the three-channel linear system model shows that we can estimate, solely from the output recordings, (1) the ratio of the transfer functions between the channels and (2) the noise spectrum for each channel. We do not need to know the transfer functions, or its accuracy as is required in the two-channel method.
Figure 4 shows the self-noise of two modern dataloggers, the Quanterra Q4120 and the NARS datalogger. The self-noise was determined with the new technique, using the vertical output of an STS-2 sensor as common input, and compared to the noise level which was measured with closed digitizer inputs. Both digitizers produce some additional noise during the digitizing process of a real input signal, and this disturbance is more pronounced in the NARS datalogger. Both digitizers have a flat noise level at higher frequencies but show a large difference in the dynamic range of about 16 dB. The self-noise levels are above the reference ambient Earth noise level, for frequencies above 1 and 8 Hz respectively, which is too high to record true seismic background noise at the quietest places in the world at high frequencies. Also the frequency at which the 1/f type of noise becomes dominant is different (0.1 Hz for NARS, 0.4 Hz for Q4120), as well as the slope of the 1/f noise. The slope for the Q4120 is modelled with 1/f 1.55 and for the NARS datalogger with 1/f 1.0.
The new 3-channel correlation technique is a novel, robust and reliable analysis technique to estimate instrumental self-noise, without a priori knowledge of the properties of the instrument. The technique is becoming the standard for manufacturers of seismic equipment and major sensor testing projects to provide accurate estimates of instrumental self-noise. From a scientific point of view the new method is important as it reveals under which conditions the interpretation of seismic data may be biased by the recording system. It is expected that this technique has to be used in studies towards a revised NLNM as to identify the contribution of instrumental noise to the NLNM.